Sin Cos Tan Values In trigonometry, sin cos and tan values are the primary functions we consider while solving trigonometric problems. These trigonometry values are used to measure the angles and sides of a right-angle triangle. Apart from sine, cosine and tangent values, the other three major values are cotangent, secant and cosecant. `sin theta =y/r` `cos theta =x/r` `tan theta =y/x` Notice that we are still defining. Sin θ as `'opp'/'hyp'`; cos θ as `'adj'/'hyp'`,. Tan θ as `'opp'/'adj'`, but we are using the specific x-, y- and r-values defined by the point (x, y) that the terminal side passes through. We can choose any point on that line, of course, to define our. The other four trigonometric functions (tan, cot, sec, csc) can be defined as quotients and reciprocals of sin and cos, except where zero occurs in the denominator. It can be proved, for real arguments, that these definitions coincide with elementary geometric definitions if the argument is regarded as an angle given in radians.

sin cos and tan are basically just functions that relate an angle with a ratio of two sides in a right triangle. Cos is adjacent over hypotenuse. And tan is opposite over adjacent, which means tan is sin/cos.

Consequently, what does Sin Cos Tan equal?

The tangent of x is defined to be its sine divided by its cosine: tan x = sin x cos x . The cotangent of x is defined to be the cosine of x divided by the sine of x: cot x = cos x sin x .

Sin, cos and tan Before we can use trigonometric relationships we need to understand how to correctly label a right-angled triangle. There are three labels we will use: Hypotenuse - The longest.

In view of this, what is the relationship between sin and tan?

Sine Function:	sin(θ) = Opposite / Hypotenuse
Cosine Function:	cos(θ) = Adjacent / Hypotenuse
Tangent Function:	tan(θ) = Opposite / Adjacent

Similarly, what is cos and sin in math?

Sine and cosine - a.k.a., sin(θ) and cos(θ) - are functions revealing the shape of a right triangle. Looking out from a vertex with angle θ, sin(θ) is the ratio of the opposite side to the hypotenuse , while cos(θ) is the ratio of the adjacent side to the hypotenuse .

Related questions and answers

What is the ratio of tan?

What is trigonometry formula?

Who invented trigonometry?

What is the formula for a 45 45 90 Triangle?

Does 5 12 and 13 make a right triangle?

Are all isosceles triangles 30 60 90?

Does 4 5 6 make right triangles?

How is sin calculated?

How do you solve A2 B2 C2?

What is Tan used to find?

Which of the following are true statements about a 30 60 90 Triangle?

Does 9 12 and 15 make a right triangle?

What are the basics of trigonometry?

How do you find a 30 60 90 Triangle?

Is this a right triangle?

What is SOH CAH TOA?

What are the 2 types of special right triangles?

• 45°; 45°; 90° Triangle.
• 30°; 60°; 90° Triangle.

What are the side lengths of a 30 60 90?

How do you find the sides of a 30 60 90 Triangle?

1. Short side (opposite the 30 degree angle) = x.
2. Hypotenuse (opposite the 90 degree angle) = 2x.
3. Long side (opposite the 60 degree angle) = x√3.

Who is father of trigonometry?

What are the 3 trigonometric ratios?

What is the 30 60 90 Triangle rule?

How do you do special right triangles?

The basic trigonometric functions include the following (6) functions: sine (left(sin xright),) cosine (left(cos xright),) tangent (left(tan xright),) cotangent (left(cot xright),) secant (left(sec xright)) and cosecant (left(csc xright).)

All these functions are continuous and differentiable in their domains. Below we make a list of derivatives for these functions.

Derivatives of Basic Trigonometric Functions

We have already derived the derivatives of sine and cosine on the Definition of the Derivative page. They are as follows:

[{{left( {sin x} right)^prime } = cos x,;;}kern-0.3pt{{left( {cos x} right)^prime } = – sin x.}]

Using the quotient rule it is easy to obtain an expression for the derivative of tangent:

[
{{left( {tan x} right)^prime } }={ {left( {frac{{sin x}}{{cos x}}} right)^prime } }
= {frac{{{{left( {sin x} right)}^prime }cos x – sin x{{left( {cos x} right)}^prime }}}{{{{cos }^2}x}} }
= {frac{{cos x cdot cos x – sin x cdot left( { – sin x} right)}}{{{{cos }^2}x}} }
= {frac{{{{cos }^2}x + {{sin }^2}x}}{{{{cos }^2}x}} }
= {frac{1}{{{{cos }^2}x}}.}
]

Sin Cos Tan Table

The derivative of cotangent can be found in the same way. However, this can be also done using the chain rule for differentiating a composite function:

[require{cancel}
{{left( {cot x} right)^prime } = {left( {frac{1}{{tan x}}} right)^prime } }
= { – frac{1}{{{{tan }^2}x}} cdot {left( {tan x} right)^prime } }
= { – frac{1}{{frac{{{{sin }^2}x}}{{{{cos }^2}x}}}} cdot frac{1}{{{{cos }^2}x}} }
= { – frac{cancel{{{cos }^2}x}}{{{{sin }^2}x cdot cancel{{{cos }^2}x}}} }
= { – frac{1}{{{{sin }^2}x}}.}
]

Similarly, we find the derivatives of secant and cosecant:

[
{{left( {sec x} right)^prime } = {left( {frac{1}{{cos x}}} right)^prime } }
= { – frac{1}{{{{cos }^2}x}} cdot {left( {cos x} right)^prime } }
= {frac{{sin x}}{{{{cos }^2}x}} }
= {frac{{sin x}}{{cos x}} cdot frac{1}{{cos x}} }
= {tan xsec x,}
]

[
{{left( {csc x} right)^prime } = {left( {frac{1}{{sin x}}} right)^prime } }
= { – frac{1}{{{{sin }^2}x}} cdot {left( {sin x} right)^prime } }
= {-frac{{cos x}}{{{{sin }^2}x}} }
= {-frac{{cos x}}{{sin x}} cdot frac{1}{{sin x}} }
= {-cot xcsc x.}
]

Table of Derivatives of Trigonometric Functions

The table below summarizes the derivatives of (6) basic trigonometric functions:

In the examples below, find the derivative of the given function.

Solved Problems

Click or tap a problem to see the solution.

Sin Cos Tan Chart

Solution.

Using the linear properties of the derivative, the chain rule and the double angle formula, we obtain:

[
{y’left( x right) }={ {left( {cos 2x – 2sin x} right)^prime } }
= {{left( {cos 2x} right)^prime } – {left( {2sin x} right)^prime } }
= {left( { – sin 2x} right) cdot {left( {2x} right)^prime } – 2{left( {sin x} right)^prime } }
= { – 2sin 2x – 2cos x }
= { – 4sin xcos x – 2cos x }
= { – 2cos xleft( {2sin x + 1} right).}
]

Solution.

The derivative of this function is

[
{y’left( x right) }={ {left( {tan x + frac{1}{3}{{tan }^3}x} right)^prime } }
= {{left( {tan x} right)^prime } + {left( {frac{1}{3}{{tan }^3}x} right)^prime } }
= {frac{1}{{{{cos }^2}x}} + frac{1}{3} cdot 3{tan ^2}x cdot {left( {tan x} right)^prime } }
= {frac{1}{{{{cos }^2}x}} + {tan ^2}x cdot frac{1}{{{{cos }^2}x}} }
= {frac{{1 + {{tan }^2}x}}{{{{cos }^2}x}}.}
]

The numerator can be simplified using the trigonometric identity

[
{1 + {tan^2}x = {sec ^2}x }
= {frac{1}{{{{cos }^2}x}}.}
]

Therefore

[
{y’left( x right) }={ frac{{1 + {{tan }^2}x}}{{{{cos }^2}x}} }
= {frac{{frac{1}{{{{cos }^2}x}}}}{{{{cos }^2}x}} }
= {frac{1}{{{{cos }^4}x}} }
= {{sec ^4}x.}
]

Solution.

Using the power rule and the chain rule, we get

[{y^prime = left( {cos x – frac{1}{3}{{cos }^3}x} right)^prime }={ left( {cos x} right)^prime – left( {frac{1}{3}{{cos }^3}x} right)^prime }={ – sin x – frac{1}{3} cdot 3{cos ^2}x cdot left( {cos x} right)^prime }={ – sin x – {cos ^2}x cdot left( { – sin x} right) }={ – sin x + {cos ^2}xsin x }={ – sin xleft( {1 – {{cos }^2}x} right) }={ – sin x,{sin ^2}x }={ – {sin ^3}x.}]

Solution.

We find the derivative of this function using the power rule and the chain rule:

[
{y’left( x right) = {left( {frac{1}{{{{cos }^n}x}}} right)^prime } }
= {{left[ {{{left( {cos x} right)}^{ – n}}} right]^prime } }
= { – n{left( {cos x} right)^{ – n – 1}} cdot {left( {cos x} right)^prime } }
= { – n{left( {cos x} right)^{ – n – 1}} cdot left( { -sin x} right) }
= {frac{{nsin x}}{{{{cos }^{n + 1}}x}}.}
]

Here we assume that (cos x ne 0), that is (x ne {largefrac{pi }{2}normalsize} + pi n,) (n in mathbb{Z}.)

Solution.

By the quotient rule,

[require{cancel}{y^prime = left( {frac{{sin x}}{{1 + cos x}}} right)^prime }={ frac{{cos x left( {1 + cos x} right) – sin x cdot left( { – sin x} right)}}{{{{left( {1 + cos x} right)}^2}}} }={ frac{{cos x + {{cos }^2}x + {{sin }^2}x}}{{{{left( {1 + cos x} right)}^2}}} }={ frac{cancel{1 + cos x}}{{{{left( {1 + cos x} right)}^cancel{2}}}} }={ frac{1}{{1 + cos x}}.}]

Solution.

Applying the power rule and the chain rule, we obtain:

[
{y’left( x right) = {left( {{{cos }^2}sin x} right)^prime } }
= {2cos sin x cdot {left( {cos sin x} right)^prime } }
= {2cos sin x cdot left( { – sinsin x} right) cdot}kern0pt{ {left( {sin x} right)^prime } }
= { – 2cos sin x cdot sin sin x cdot}kern0pt{ cos x.}
]

Sin Cos Tan Examples

The last expression can be simplified by the double angle formula:

[
{2cos sin x cdot sin sin x }
= {sin left( {2sin x} right).}
]

Consequently, the derivative is

[{y’left( x right) }={ – sin left( {2sin x} right)cos x.}]

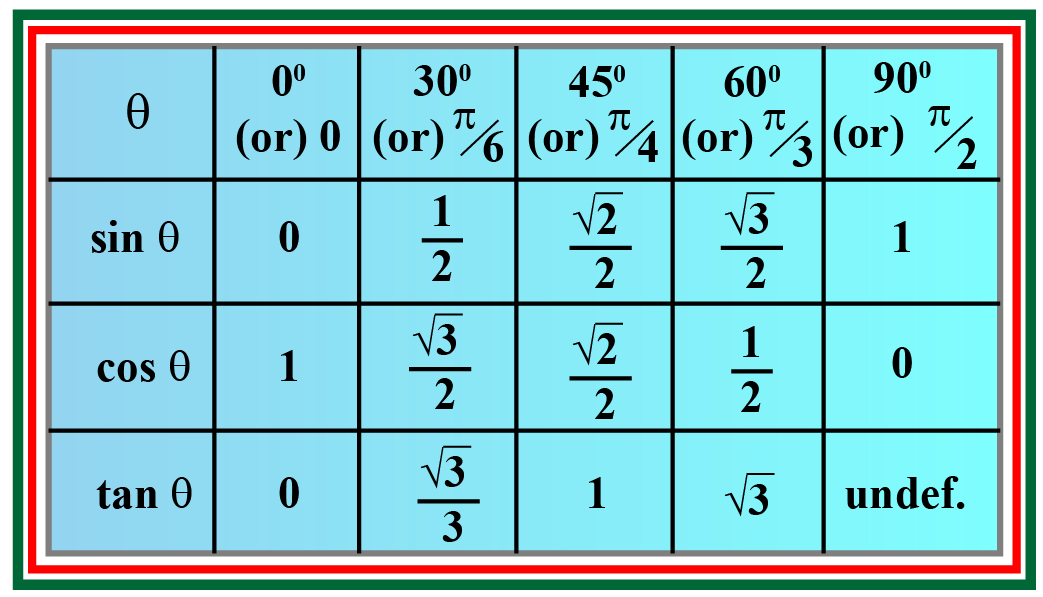
Solution.

Using the product rule, we can write:

[{y^prime = left( {xsin x + cos x} right)^prime }={ left( {xsin x} right)^prime + left( {cos x} right)^prime }={ x^primesin x + xleft( {sin x} right)^prime + left( {cos x} right)^prime }={ 1 cdot sin x + x cdot cos x + left( { – sin x} right) }={ cancel{sin x} + xcos x – cancel{sin x} }={ xcos x.}]

Sin Cos Tan Triangle

Unit Circle Sin Cos Tan